There is uncertainty everywhere: in simple decisions like shooting a basketball or complex ones like analyzing a data set. Uncertainty isn’t random, however, and you can usually predict, within a certain amount, how accurate your estimate will be. The confidence interval formula is a way to calculate uncertainty in a given experiment. This article will detail the confidence interval formula, why it’s important, and how to use it.
Confidence Interval Formula and Definition
The confidence interval formula is an equation that, given a predetermined confidence level, provides a range of values that you expect your result to fall within if you conduct the experiment again.
The most common confidence level is 95%, but other levels such as 90% and 99% can also be used. If you use 95%, for example, you think that 95 out of 100 times, the estimate will fall within the parameters of the confidence interval.
The formula for the confidence interval looks like this:
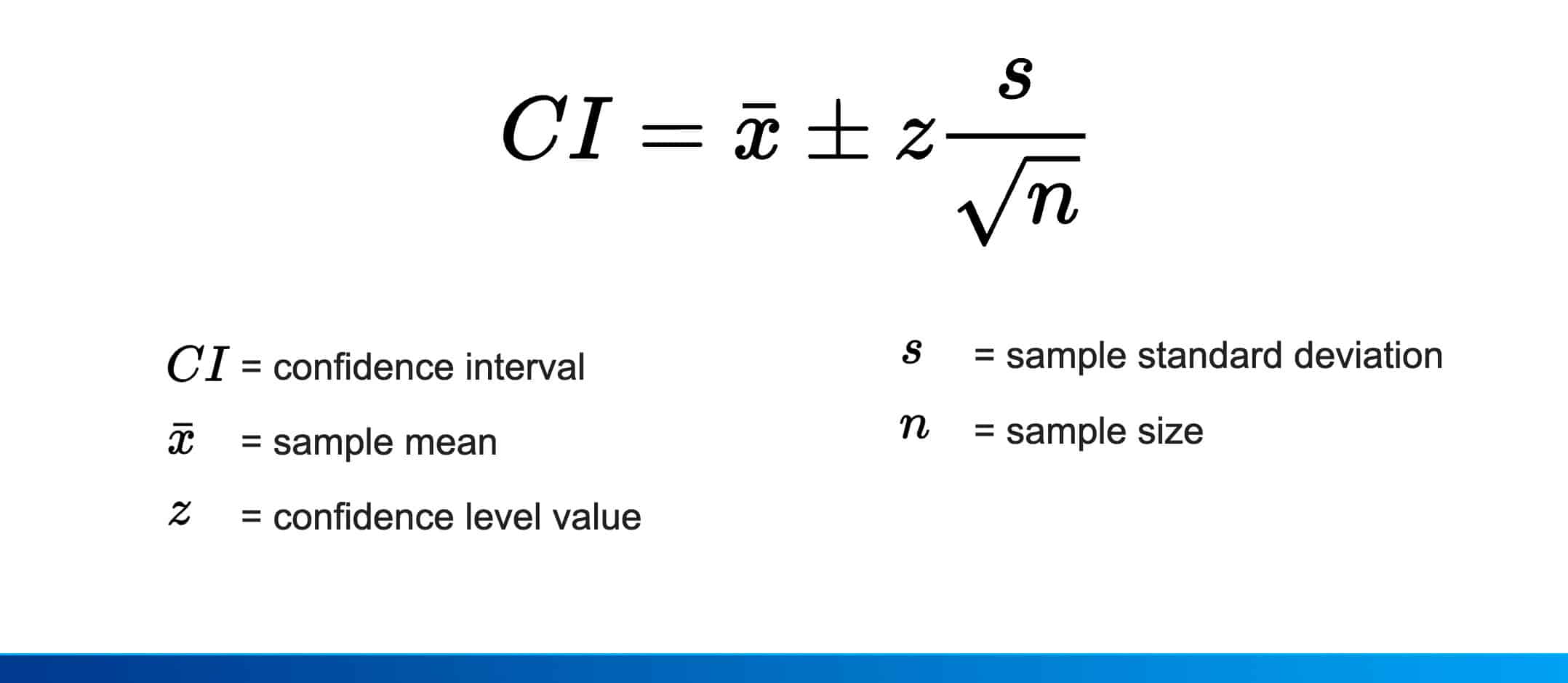
The confidence level is set by the alpha value used in the experiment and represents the number of times (out of 100) you think the expected result will be reproduced. If the alpha was .1, then the confidence level will be 1-.1=.9, or 90%.
The overall confidence interval represents the average of your estimate plus or minus the variation within the estimate. This is the expected range of values, with a certain amount of confidence, your values to fall into.
Why Is the Confidence Interval Formula Important?
Establishing a confidence interval is important in terms of probability and certainty. The formula laid out above allows survey conductors to estimate how well results will be reproduced and what they expect with a high degree of accuracy. Setting clear expectations is an important part of understanding how well a survey is understood, acted on, and how accurate an initial set of data might be. Additionally, setting expectations can be helpful when conducting a customer needs analysis.
The confidence interval formula is also helpful for establishing confidence in a given audience. When conducting surveys and outreach with your customers, it can be useful to understand what they think and how they respond. The confidence interval allows you to use this information to accurately predict how they should respond to future experiments and will tell you if something changes in the audience.
Confidence Interval Formula Use Guide & Example
Here is a step-by-step guide for using the confidence interval formula. For this example, we will use an imaginary sample of people shooting 100 free throws.
Find the Average Result
The first piece of information you need is the sample mean. This is the average result across all participants. To find this, add up all the scores and divide them by the number of participants.
Our sample for shots made is 75, 80, 75, 80, 90, 75, 85, 75, 90, 80. Adding these up and dividing by the total shooters (10) gives us 80.5. This means that across all shooters, the average score was 80.5. The confidence interval will calculate the certainty that the next experiment will score the same average amount of shots.
Calculate Standard Deviation
After finding the sample average, you need to calculate the standard deviation. This will be the difference from the average for the sample size. To find the standard deviation, you must subtract the sample mean from each individual result and square each answer. Then, add them all up, and take the square rook of that number. This will be the sample standard deviation.
For our example data set, this looks like: (75 – 80.5)² + (80 – 80.5)² + (75 – 80.5)² + (80 – 80.5)² + (90 – 80.5)² + (75 – 80.5)² + (85 – 80.5)² + (75 – 80.5)² + (90 – 80.5)² + (80 – 80.5)² = 30.25 + 0.25 + 30.25 + 0.25 + 90.25 + 30.25 + 20.25 + 30.25 + 90.25 + 0.25 = 322.5 ÷ 10 total shooters = 32.25.
Find Standard Error & Margin of Error
You can now use the sample mean and standard deviation to calculate the standard error of your study. This number will represent how closely the sample represents the total population. In our example of free throws, you calculator the standard error by dividing the standard deviation by the size of the study: 32.25 / 10 = 3.225.
After figuring out the standard error, you can easily calculate the margin of error. This tells you how confident you can be when conducting the same experiment for the total population. A larger margin of error will mean less confidence in reproducing the results. To find this, multiply the standard error by two. For our data, this looks like: 3.225 x 2 = 6.45.
Plug in Your Numbers
Once you have your numbers, you can plug them into the formula and calculate your confidence interval. We will assume that the Z-value is 95% and, therefore, 0.95.
Confidence interval (CI) = ‾X ± Z(S ÷ √n) = 80.5 ± 0.95(32.25 ÷ √10) = 80.5 ± 0.95(32.25 ÷ 3.16) = 80.5 ± 0.95(10.21) = 80.5 ± 9.70 = 90.2, 70.8.
Analyze the Results
The confidence interval formula determines if your results are likely to be repeated for the total population of your sample. Higher confidence shows a higher probability of repetition, while lower confidence shows a lower likelihood of seeing the same results. With these numbers, you can get an accurate picture of the boundaries of expected results when you conduct your experiment again. With that, you can analyze population changes and predicted data.
Our confidence interval for people shooting free throws was between 90.2 and 70.8 free throws made. This means the average amount of shots made should fall between these two values (with 95% confidence) for the whole population.
Conclusion
With the confidence interval formula, you can accurately predict where people will land based on previous results and your estimated confidence. This can help predict many things, from future data to population changes, and hopefully, this guide helped uncover some important insights for your next experiment.
In QuestionPro, our reports make analysis simple. From real-time shareable dashboards to our complete suite of analytics, we help you turn data into decisions in a flash. Start today!
